Test dyna12 Description sheet

Test name

Calculation type

Finite element type

Topic

The launcher is submitted to an axial load during a limited period. Considering rigid body movements and vibration of the structure, the displacement history is searched for the bottom point.

Goal

Reference

Version

Model description

Results

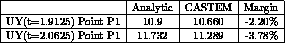
*           Test Dyna12.dgibi: Jeux de données        *
*           ---------------------------------         *
*                                                     *
GRAPH='N';                                                                      
SAUT PAGE ;                                                                     
*******************************************************
*   REPONSE TRANSITOIRE D 'UNE FUSEE -METHODE DIRECT 
*******************************************************
*
* DESCRIPTION DU PROBLEME
* -----------------------
*
* UNE FUSEE EST SOUMISE A UN CHARGEMENT AXIAL POUR UNE 
* DUREE LIMITEE. IL S'AGIT DE TROUVER LES HISTORIQUES 
* DE DEPLACEMENTS AUX EXTREMITES ET AU MILIEU DE LA 
* FUSEE CONSIDERANT LE MOUVEMENT DE CORPS RIGIDE ET 
* LA VIBRATION DE LA STRUCTURE .
*
* REFERENCE :
* ----------
*
* J. S. PRZEMIENIECKI,THEORY OF MATRIX STRUCTURAL 
* ANALYSES (NEW YORK: MCGRAW-HILL BOOK CO.,1968)P.340  
*
*******************************************************
OPTION  ECHO 0 ;
OPTIO DIME 3 ELEM SEG2 ;
*
*  MAILLAGE 
*
 P1 = 0. 0. 0. ;P2 = 0. 70. 0. ;P3 = 0. 140. 0. ;
 L1 = D 1 P1 P2 ; L2 = D 1 P2 P3 ;LTOT = L1 ET L2 ;
*
*  MODELE 
*
 MOD1 = MODELE LTOT MECANIQUE ELASTIQUE BARR ;
*
*  CARACTERISTIQUES MATERIELLES ET GEOMETRIQUES 
*
 MAT1 = MATER MOD1 YOUN 1.E4  NU 0.3 RHO 0.1 ;
 CAR1 = CARAC MOD1 SECT 1. ;
*
*  MATRICE DE RIGIDITE 
*
 RIG1 = RIGIDITE MOD1 (MAT1 ET CAR1) ;
*
*  MATRICE DE MASSE 
*
 MAS1 = MASSE MOD1 (MAT1 ET CAR1)  ;
*
* CHARGEMENT  
*
LI1 = PROG 0. 0.0125 1.0125 1.025  3. ;
LI2 = PROG 0. 1. 1. 0.  0.   ;
F1 = FORCE (0. 100. 0.) P1   ;
EV = EVOL MANU T LI1 F(T) LI2 ;
CHA1 = CHAR 'FORC' F1  EV ;
*
*  PREPARATION DE LA TABLE DE DONNEES POUR DYNAMIC 
*
LIS1 = PROG 1.9125 2.0625  ;
TAB1 = TABLE ;
TAB1.'DEPL' = MANU CHPO LTOT 3 UZ 0. UY 0. UX 0.  ;
TAB1.'VITE' = MANU CHPO LTOT 3 UZ 0. UY 0. UX 0. ;
TAB1.'CHAR' = CHA1 ;
TAB1.'RIGI' = RIG1 ;
TAB1.'MASS' = MAS1 ;
TAB1.'FREQ' = 20. ;
TAB1.'INST' = LIS1 ;
TAB2 = DYNAMIC TAB1 ;
*
* COMPARAISON AVEC LES RESULTATS ANALYTIQUES 
*
 DEP1 = TAB2 . 1 . DEPL ;
 U1 = EXTR DEP1 P1 UY ; U1TH = 10.89972 ;
 ERR1 = ((U1 - U1TH) / (ABS U1TH)) * 100. ;
 DEP2 = TAB2 . 2 . DEPL ;
 U2 = EXTR DEP2 P1 UY ; U2TH = 11.73228  ;
 ERR2 = ((U2 - U2TH) / (ABS U2TH)) * 100. ;
MESS ' DEPLACEMENT VERTICAL DE LA BASE    T= 1.9125'  ;
'MESS''THEORIE  'U1TH'CASTEM  'U1 '%ERREUR  'ERR1  ;
MESS ' DEPLACEMENT VERTICAL DE LA BASE    T= 2.0625'  ;
'MESS''THEORIE  'U2TH'CASTEM  'U2 '%ERREUR  'ERR2  ;
*
SI ((MAXI ABS (PROG ERR1 ERR2 )) < 5);
ERRE 0;
SINON;
ERRE 5;
FINSI;
FIN ;

Test dyna12 Comments

1. Load as a function of time

   LI1 = PROG 0. 0.0125 1.0125 1.025 3. ;
   LI2 = PROG 0. 1. 1. 0. 0. ;
   F1 = FORCE (0. 100. 0.) P1 ;
   EV = EVOL MANU T LI1 F(T) LI2 ;
   CHA1 = CHAR 'FORC' F1 EV ;
   The operator CHAR builds an object of type CHARGEMENT, which contains spatial and temporal descriptions of the load :
   - the spatial description is given by the object F1,
   - the temporal description is given by the object EV which is called evolution (built by the operator EVOL, EV describes the load (object LI2) as a function of the time (object LI1)).

2. Use of DYNAMIC subroutine

   * INPUT DATA FOR DYNAMIC SUBROUTINE
   **
   LIS1 = PROG 1.9125 2.0625 ;
   TAB1 = TABLE ;
   TAB1.'DEPL' = MANU CHPO LTOT 3 UZ 0. UY 0. UX 0. ;
   TAB1.'VITE' = MANU CHPO LTOT 3 UZ 0. UY 0. UX 0. ;
   TAB1.'CHAR' = CHA1 ;
   TAB1.'RIGI' = RIG1 ;
   TAB1.'MASS' = MAS1 ;
   TAB1.'FREQ' = 20. ;
   TAB1.'INST' = LIS1 ;
   TAB2 = DYNAMIC TAB1 ;
   

   The operator DYNAMIC carries out step by step dynamic computations, using the Newmark centered algorithm. All required data are given in the object TAB1 defined by the following indexes :
   -'DEPL' : initial displacements,
   -'VITE' : initial velocity which components are the same as components of initial displacements,
   -'CHAR' : the load (as a function of time),
   -'RIGI' : the rigidity matrix,
   -'MASS' : the mass matrix,
   -'FREQ' : the cut off frequency which determines the time step,
   -'INST' : the list of moments to save.
   The value of time step will be equal to 0.25 divided by the cut off frequency (in this case 20 Hz) which gives a step of 0.0125 sec.
   

3. Description of DYNAMIC result

   DEP1 = TAB2 . 1 . DEPL ; U1 = EXTR DEP1 P1 UY ;
   DEP2 = TAB2 . 2 . DEPL ; U2 = EXTR DEP2 P1 UY ;
   The object created by DYNAMIC subroutine is a table which contains as many tables as saved moments. Here TAB2 is composed of two tables because the list TAB1.INST contains two moments : TAB2 . 1 and TAB2 . 2.
   Each table has three indexes :
   - 'TEMP' : the value of the moment
   - 'DEPL' : the displacement
   - 'VITE' : the velocity
   TAB2 . 1 . DEPL is a displacement field. A value can be extracted by using EXTR operator and specifying the desired point and the required component.